-
Sometimes you run into people who want to brush up on their geometry. If you are one of these people and if the reason you want to brush up on geometry is because you use it in your career or in a hobby, Euclidean geometry is probably not the type of geometry you want. You would be hunting for the geometry of measurement ... an applied type of geometry.
While applied geometry is derived from Euclidean geometry, Euclidean geometry also puts a lot of emphasis on derivations and proofs. It is a formal logical system.
-
The famous Italian Renaissance artist, Raphael, was commissioned to decorate the Apostolic Palace of the Vatican with frescoes, or related mural paintings. One of his most acclaimed paintings is "School of Athens," in which he included many famous philosophers and figures.
Euclid is conjectured to be one of the figures in the painting, along with his students and his mentor, Aristotle. For a virtual tour of Raphael's Rooms at the Vatican, visit the Vatican Museums.
-
Here is Euclid's 5th Postulate:
- Parallel Postulate At most one line can be drawn parallel to a given line through a given point not on this line.
Here is one way to write it as a syllogism.
• Premise: Given a line and a point not on the line, there is at most one line that passes through the point and is parallel to the given line. • Premise: You are given a line and a point not on the line. • Conclusion: There is at most one line that passes through the point and is parallel to the given line. 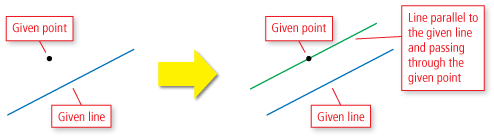
-
Comments (1)
These comments are not screened before publication. Constructive debate about the information on this page is welcome, but personal attacks are not. Please do not post comments that are commercial in nature or that violate copyright. Comments that we regard as obscene, defamatory, or intended to incite violence will be removed. If you find a comment offensive, you may flag it.
When posting a comment, you agree to our Terms of Use.Showing 1 commentsSubscribe by email Subscribe by RSSRon Larson (author)1 decade ago |I debated with myself as to whether I should include Examples 3 and 4 in the book. Finally, I decided that the examples make an important point. That point is that it is possible to have two logical systems, each of which is a valid model of "part of the universe" and yet the two systems are incompatible with each other.0 1





